1 INTRODUCTION

The Big-bang model assumes that the universe has been formed from interacting particles, which have associated to form nuclei, then atoms, then molecules, then more and more extended and complex objects, giving rise to the whole tree of living beings, from bacteria to animals presenting some consciousness. A strict reductionism cannot explain how this evolution has been possible, how new properties have been able to emerge and jump the energetic gaps between levels.

Our aim is to propose a mathematical model, based on Category Theory (cf. Mac Lane [18]), which justifies an "emergentist reductionism" in the sense of Mario Bunge [3]. It presents results we have developed in a series of papers since 15 years, a summary of which can be found in our Internet site (indicated above). It rests on the Multiplicity Principle (generalizing the "Degeneracy Principle" of Edelman [9]) which asserts the existence of complex objects, called multifold objects, which may balance between several configurations. If a category K satisfies this Principle, we prove that any complexification of K also satisfies it, and explain how it entails the emergence of more and more multifold objects whose properties are not reducible to those of their lower order constituents, though they depend in a precise manner of the whole of the lower level (whence a kind of controlled delocalization).

This model can be applied to natural systems because the laws of Quantum Physics ensure that the MP is satisfied in the category of particles and atoms, so that it is also satisfied in the systems which have evolved from this category. It gives a precise sense in which properties of quantum processes are at the root of the emergence of higher and higher processes up to consciousness, as many authors have hypothesized (e.g., Hameroff and Penrose [16], Pribram [23],…)

Section 2 is devoted to the general mathematical model; the categorical tools are only briefly defined and we send back to Section 4 for precise definitions of the words followed by *. It is then applied to the evolution of natural systems such as biological and neural systems, modeled by the Memory Evolutive Systems (MES) which are particular anticipatory autonomous systems (in the sense of Rosen [24], cf. also [7]).

In Section 3, cognitive systems, modeled by the MES of neurons, are investigated in more details, emphasizing the signification of the MP in this case. In particular, we describe how higher animals become able to record their experiences and classify their records to develop a semantics. The existence of a semantics allows the development, from birth on, of a sub-system of the MES, the archetypal core AC, whose multifold objects integrate all the sensorial, emotional, motor experiences and the basic strategies associated to them; its links are strong, quickly activated, gradually strengthened and thus are the basis of the notion of self..

We characterize consciousness as a process which, relying on this AC, integrates the temporal dimensions: (i) A new event will start a semiotic search in the AC that, through balances between configurations of multifold objects, leads to the formation of a 'holist' extended landscape, whose objects are the temporal perspectives of the event and of its internal reflections; (ii) this extended landscape allows to perform a retrospection process in the lower levels of the near past to find, by abduction, the causes of the present event, and (iii) a prospection process (oriented toward the future) to select long term strategies to respond in the most adaptive way.

2 A GENERAL MODEL FOR EMERGENCE

2.1 Evolutive Systems

The state of a system, such as a biological, social or neural system, at a time t is modeled by a category*: its objects represent the components (of any level) of the system, and the morphisms (called links) their interactions in the system around this time. These links may represent more or less stable structural relations such as causal or topological relations (e.g., desmosomes between adjacent cells), channels transmitting information, spatial or energetic constraints, or temporary interactions between two components. The composition rule associates to 2 successive links their cumulative interaction; it is associative and so it allows to distinguish among the paths of successive links those which correspond to equivalent cumulative interactions.

The changes between successive states of the system are represented by partial functors* between the corresponding categories, so that the system is represented by an Evolutive System* K, that is by a sequence of categories representing its states K_t at successive dates t of a (finite or infinite) timescale T and partial functors between them called transitions (Ehresmann & Vanbremeersch [10]). A sub-system* of K is an evolutive system such that its timescale S is included in T, for each date s in S its category is a sub-category of K_s and its transitions are restrictions of those of K.

2.2 Complex Objects

In natural systems, the objects are partitioned into different complexity levels, each level satisfying its own rules. There are intralevel links, but also interlevel links. An object of level n+1 is an aggregate of objects of level n, bound by strong interactions between them which generate their cohesion in the aggregate. For instance in an atom, the nucleus and the electrons are united through the attraction forces between electrons and protons, repulsion forces between the electrons and inside the nucleus; thus the atom has an internal organization depending on its electronic conformation.

How can we model such a hierarchical organization in a category? The idea is to use the well-known colimit operation (Kan [17]), so that an aggregate N will be modeled by the colimit of a pattern of linked objects representing its internal organization.

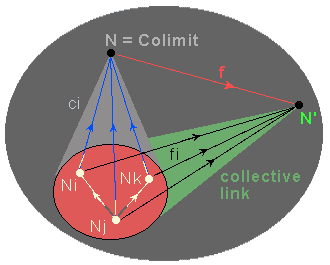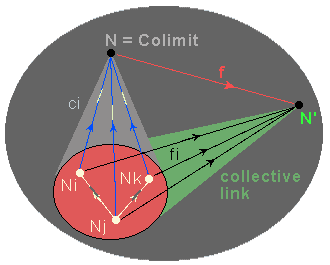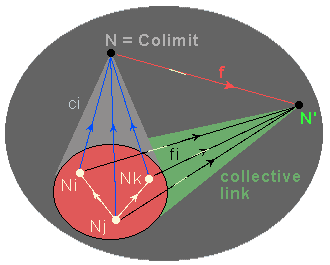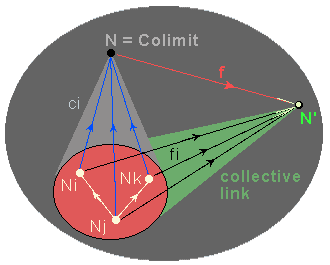
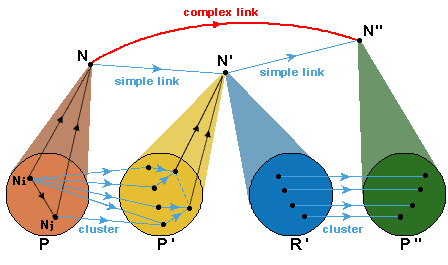
A pattern* P is defined as a family of objects N_i of the category and some distinguished links between them. A collective link* from P to an object N' is a family of links (f_i: N_i ® N') correlated by the distinguished links of the pattern. Collective links model coherent interactions (constraints, energy, or information transfer) effected by all the N_i acting in cooperation along their distinguished links, which could not be realized by the objects of the pattern acting individually.

A pattern P may have collective links toward several objects. P has an aggregate if, among those collective links, there is an 'optimal' one, i.e. a (c_i: N_i ® N) through which all the others factorize. In categorical terms, this means that N is the colimit* of P; we also say that N binds P, or is a glue (Paton [22]) of P ; or, seen in the opposite direction, that P is a decomposition of the complex object N. The colimit has localized properties in being an objectual representation of the pattern it binds, that so acquires a dynamical stability; but it has also a 'global' implication for its surround through its 'universal' property of factorizing every collective link.

A colimit must not be confused with the simple sum* of the objects of the pattern (without the distinguished links), which does not take into account their coherent behavior. For instance, in the category of quantum objects (particles up to atoms) and their interactions, a superposition or an entanglement is a colimit, while a mixed state in just a sum.

2.3 Multiplicity Principle

Some interactions between complex objects can be directly transposed from interactions distributed among the lower level constituents of these objects; in energy terms they don't add anything new to what is known at this lower level. In the categorical model, they are modeled by 'simple links': if N is the colimit of P and N' a colimit of P', a (P,P')-simple link* from N to N' is a link which binds a cluster of links from P to P'; such a cluster* is a family of links from objects N_i of P to objects N'_j of P', well correlated by the distinguished links of the two patterns so that they collectively send coherent information to P'.

Roughly, a simple link 'institutionalizes' the cluster, without adding any information which is not accessible at the level of the patterns. For instance, in Embryology, the induction of a population of cells by another corresponds to the formation of a simple link. A composite of simple links binding adjacent clusters is a simple link.

However in some categories, there exist links which are not simple though they are the composite of simple links, but binding non-adjacent clusters; they are called complex links*. This is possible if there exist objects N' which are the colimit of two non-equivalent patterns, say P' and R'. Such objects are called multifold objects*, and the balance between P' and R' is called a complex switch. During the evolution of the system, it allows that P' and R' exchange their functional role, though there is no localized interaction distributed among their constituents.

We will see that the existence of complex switches has important consequences for the problem of emergence. Thus we have introduced (Ehresmann & Vanbremeersch [12]):

Definition: A category satisfies the Multiplicity Principle (MP) if some of its objects are multifold, that is if there exist non-equivalent patterns admitting the same colimit. An evolutive system satisfies the MP if its state categories satisfy the MP.

If the category satisfies the MP, there exist complex links. A complex link from N to N" is obtained as the composite of a (P,P')-simple link from N to a multifold object N' with a (R',P")-simple link from N' to N", where P' and R' are two non-equivalent decompositions of the multifold object N'. It represents properties which do not depend only on the interactions between the objects of the decompositions P and P" of N and N", but also, in a more complicated manner, on the properties of the decompositions P' and R' of the intermediate multifold object N' and on the existence of a complex switch betwen P' and R' (e.g. representing an energy transformation). Thus it has global emerging properties with respect to the decompositions of N and N" given by P and P", these new properties depending on the lower level structure, but taken in its globality and not only through the constituents of N and N". A composite of complex links is a complex link (or in rare cases, a simple link).

The laws of Quantum Physics ensure that the 'quantum system' (modeling the system) of quantum particles and atoms and their interactions satisfies the MP. Indeed, an atom is the colimit of any of its electronic configurations in atomic orbitals, and there are complex switches between the configurations related to different energy levels. From that, we will deduce (cf. Section 2.6) that the MP is also satisfied in natural systems, since they are obtained from sub-systems of the quantum system by the complexification process. In such concrete systems a complex switch generally corresponds to an energy transformation; it can be seen as a random fluctuation in the internal organization of an object which does not modify its functionality at the higher level: several microstates lead to the same macroequilibrium. An example of such a switch is the passage between genotypes with differing alleles leading to the same phenotype.

2.4 Hierarchical Systems

A hierarchical system is an Evolutive system in which each category is hierarchical, that is its objects are partitioned into a sequence of complexity levels 0, 1,..., m, so that each object N of level n+1 is the colimit of at least one pattern of linked objets N_i of level n. In this case, since N is the colimit of a pattern P of linked objects N_i of level n and each N_i is the colimit of a pattern P_i, the object N is the 2-iterated colimit of (P,(P_i)), and (P,(P_i)) is called a ramification of N of length 2. The ramification represents an internal organization of N specifying two levels, which determines in 2 steps the links of N to the other objects of the category.

More generally, we inductively define a k-iterated colimit and a k-ramification of an object A of level > k. Roughly, A has a kind of fractal structure, whose components at each intermediate step are themselves ramified, but moreover with correlation between these ramifications introduced by the 'horizontal' distinguished links between the components at each level.

If the MP is satisfies, a multifold object A has a multiplicity of ramifications which make this structure very flexible. Indeed, if we think that a decomposition of A attributes particular values ('variables') to some characteristic features of A, as the slots in a frame (in the sense of Minsky [21]), a k-ramification amplifies the choice since successive choices can be done at each of its k steps. The number of non-equivalent ramifications of A arriving to a lower level k gives a measure of the flexibility of A, in the sense of the number of its functional internal organizations down to this level; it could be called the k-entropy of A

2.5 Emergentist Reductionism

In a hierarchical system, the level of an object N is not a good warrant of its 'real' complexity; this is measured by the order of N, which is the smallest p such that there exists a pattern of linked objects of level p admitting N as its colimit. And N is said to be q-reducible for each q less or equal to its order.

By definition, any object of level n+1 is n-reducible. When is it p-reducible, for some p < n? To answer, we must more precisely classify the links: A link between two objects N and N' of level n+1 is n-simple if it binds a cluster between two patterns of level less or equal to n. It is n-complex if it is the composite of n-simple links binding non-adjacent clusters, without being itself n-simple. Moreover, there might also exist links which are nor n-simple nor n-complex, and which represent constraints unrelated to the lower levels.

Then the following result is proved (Ehresmann & Vanbremeersch [12]):

Theorem. An object N of level n+1 is (n-1)-reducible if it admits a decomposition into a pattern of objects N_i of level n in which the distinguished links are (n-1)-simple links binding adjacent clusters between the N_i. Otherwise, N might not be (n-1)-reducible. This result extends to lower levels.

This theorem demonstrates the limits of the strict reductionist program which assumes that any object is reducible, in one step, to the lowest level, i.e., is of order 0. However in most natural hierarchical systems, there is a kind of reduction to lower levels, but in several steps and with the emergence at each level of new properties reflecting holistic properties of the preceding level. Such systems are k-based, for some k, in the sense that the links of level n+1 are n-simple or n-complex, for each n > k, i.e., no external constraints are added in the passage from the level k up. In such a system not only each object is stepwise reconstructed from the level k up through a ramification (that is true in any hierarchical system), but the links can also be stepwise reconstructed since they either bind clusters of the next lower level, or are composites of such. However this reconstruction depends not only on the 'local' properties of the components of level k of the object or link (as required by the reductionist program), but also on the global structure of each successive level. Thus there is really emergence of new properties with respect to the components of the object.

Indeed, we have seen above that the properties of a k-complex link from N to N" depend not only on those of the patterns N and N" bind but also on those of the intermediate multifold objects intervening in its construction with complex switches; thus emerge from the global structure of level k.

Now let A be a level k+2 object. It can be reconstructed from level k as a 2-iterated colimit of a ramification (R,(P_i)); but, if some of the links of R are complex, it follows that they also impose on A properties emerging from the global structure of level k, and not reflected from the sole local properties of the lower components of A.

We can speak of an 'emergentist reductionism' (making precise the notion introduced by Mario Bunge [3]). In particular, for 0-based systems (i.e. if k = 0) the non-linear language of the system will be entirely decoded given the primitive terms, i.e., the objects of level 0 and their interaction, and the 'syntax' which indicates how they are bound together to progressively construct the higher levels objects and links in several steps, with, at each level, emergence of new properties depending on the global structure of the preceding level.

Such hierarchical systems can be constructed by the following

2.6 Complexification Process

This situation occurs in natural evolutionary systems, which are based on sub-systems of the quantum system. In the evolution/development of a natural system, the change of states is regulated by the process of "birth, death, scission, collision" (Thom [25]); for a cell, endocytosis, exocytosis, breakage and synthesis of macromolecules. This is modeled by the process of complexification of a category with respect to a strategy.

A strategy S on a category K is the data of: external elements 'to absorb'; some objects or links of the category 'to suppress', or to decompose; patterns without a colimit 'to bind together', so that a colimit be added; cones 'to transform into colimit-cones', so that the vertex of the cone becomes a colimit of its basis.

The complexification is explicitly described (Ehresmann & Vanbremeersch [10]): Its objects are: all the objects of K not suppressed by S, the elements to absorb and, for each pattern P to bind, a new object colim P becoming its colimit, which emerges as the integration of the pattern into a higher order unit. Its links are of two types: simple links binding clusters between patterns of K, and complex links which are composites of simple links binding non-adjacent clusters. In the terms of Farre [14], we can think of P as the internal causal cycle, while the appearance of colim P separates it from its context and gives it an objectual character.

We have seen that the emergence of new properties requires the existence of multifold objects. Now we have:

Theorem. If K satisfies the MP, so does any complexification of K.

The complexification process can be iterated, and, if K satisfies MP, successive complexifications lead to the emergence of a hierarchy of complex objects of increasing complexity order, with emerging properties at each level. Thus the MP explains how a 'real' emergence can occur.

Corollary. The MP is satisfied in the quantum system and in the natural systems obtained by successive complexifications of its sub-systems.

We have seen in Section 2.3 that Quantum Physics implies that the MP is satisfied in the quantum system. From the above Theorem it follows that the MP is also satisfied in successive complexifications of its sub-systems, so that they lead to a hierarchy of more and more complex objects, modeling the evolution of the whole physical universe. Most natural systems, in particular biological, neural or social systems, are obtained in this way.

Remark that, in the quantum system, a complex switch results from a quantum process. In successive complexifications, there are still complex switches. As we have seen above, they emerge from the whole structure of the lower levels, hence are based on the properties of quantum processes, such as superposition and non-localization. But that they can be reconstructed from such a base does not mean that multifold objects 'have' quantum properties; in fact their macro-properties are classical. So we differ from the interpretation of Aerts et al. [1] who speak of "quantum-mechanical properties" for cognitive interactions. In fact, their examples are easily translated into saying that the objects they consider (e.g. the concept cat) are multifold, whence the properties they describe.

3 COGNITIVE SYSTEMS UP TO CONSCIOUSNESS

Autonomous natural systems, such as biological, neural or social systems, are obtained by successive complexifications of sub-systems of the quantum system, as described above, so that they satisfy the MP. These systems are open, self-regulated, able to record their experiences in a flexible way and to adapt to their environment by recognizing features already met and recalling what are the best strategies for coping with. In this sense they are "anticipatory systems" in the sense of Rosen [24], developed by many authors, in particular Dubois (cf. [7]). However we don't use this terminology but prefer to look in the other way. In [11], we have introduced a model for these systems, called Memory Evolutive Systems (MES).

3.1 MES

The complexification process supposes first that a strategy has been selected on the category to complexify. In autonomous systems, the strategies will be selected in an internal manner, taking into account the information coming from the environment and from the internal state. For this, we suppose that there exists a net of internal organs of regulation, called CoRegulators (CR). And these CRs will participate in the development of a central Memory to record the different experiences.

More precisely, a MES is a hierarchical evolutive system the architecture of which is a compromise between a parallel distributed system or multi-agents system, and a hierarchical associative net. It has a hierarchical sub-system, called the Memory and noted Mem which develops in time to record and store the different situations it encounters and the strategies used, so that they might be recalled later on. Its dynamics is modulated by the cooperation and/or competition between a net of internal sub-systems, the CoRegulators (CR).

Each CR operates, at its own complexity level and with its own temporality, a stepwise trial-and-error learning process, using its differential access to the central Memory at the development of which it participates. At a given time, a CR can acquire only partial information on the system and its situation, more or less restricted depending on the complexity level of its objects (called agents); for instance, lower CRs may perceive only some attribute (such as the color or the shape) while higher associative CRs have a larger base, possibly controlling several lower CRs. These informations form its actual landscape*.

We can think of a CR as an observable, the landscape of which is its observation space containing the particular measures it can make. But the CR does not only 'measure'; it has also an 'active' role of selection of a strategy to react to the information it has received. This could be compared with the notion of a transaction in the Transactional Interpretation of Quantum Physics (Cramer [5]): the CR sends a "question" (analog of the "offer wave") to the memory which activates similar former experiences and the strategies then used with their results, these are reflected in the landscape ("confirmation wave"), eventually compared, and the "transaction" is achieved by the selection of a strategy and the sending of the corresponding commands to effectors. If the command concerns a multifold object, its realization may choose any one of its decompositions (to be compared with the collapse of a wave packet). Which decomposition is used will depend on the context, e.g. on the strategies simultaneously selected by the other CRs.

Indeed, each CR operates independently on its landscape, but they partake the common resources of the system. Thus the strategies of the different CRs are reflected to the whole system where they must be made coherent and preserve the structural and temporal constraints of each CR. This is achieved through an equilibration process, called the interplay among their strategies. It may result in fractures for some CRs, if there are discrepancies which cannot be resolved.

This interplay is heavily dependent on the existence of complex switches, which allow to realize the various commands of a strategy by selecting among their ramifications those which give the most coherent ensemble. It generates a dialectics between CRs heterogeneous with respect to their temporality and complexity, relying on functional loops, that can account for the emergence of adaptive complex phenomena.

3.2 MES of neurons

Let us now concentrate on cognitive systems, corresponding to the development and functioning of the nervous system of a higher animal. It is modeled by the MES of neurons, obtained by successive complexifications of the category of neurons defined as follows: its objects are the neurons, the links are classes of synaptic paths between neurons, two such paths being identified if they are functionally equivalent, i.e. if they have the same strength. As this category is itself the complexification of a sub-system of the quantum system, it satisfies the MP; hence so does the MES of neurons obtained by its successive complexifications. Let us see what it means in this case.

The response of a neural system to a simple stimulus is the activation of an individual, specialized neuron; for instance, in the visual area, there exist 'simple cells' representing a segment of a given direction, and 'complex cells' representing a particular angle. But more complex stimuli, except for some exceptions (e.g., a neuron activated by a hand holding a banana for a monkey), do not have their own 'grand-mother neuron'. The development of neuronal imaging seems to confirm this 'associationist' idea: complex perceptual stimuli, or motor programs, are represented by the short-lived synchronized firing of a specific assembly of neurons. And learning would consist in the formation of such synchronous assemblies, through the strengthening of synapses between their neurons, following the rule already proposed by Hebb [15]: a synapse between two neurons is strengthened if the two neurons fire at the same time, and depressed if one fires while the other does not.

An assembly of neurons is represented by a pattern P in the category of neurons Neur. The synchronization of this assembly will be modeled by the emergence of a colimit of this pattern in a complexification of Neur; this colimit, which operates as a unique 'higher order neuron' integrating the synchronous assembly, will be called a category-neuron (or simply a cat-neuron). The construction of the complexification determines what are the 'good' links between cat-neurons, hence between synchronous assemblies of neurons, thus giving an answer to the 'binding problem' studied by neuroscientists (cf. von der Malsburg [19]). The MP being satisfied, the same cat-neuron can represent different synchronous assemblies, which explains the flexibility of the memory. And it allows to define, by iterations of the complexification process, more and more complex cat-neurons, representing assemblies of assemblies of neurons, ... corresponding to the development of higher order mental objects and cognitive processes.

Thus the general results of Section 2 show in which precise sense cognitive processes of any level are based on quantum processes. Remark that several authors have proposed that quantum processes are responsible for cognitive processes, but without describing exactly by which means. In fact their theories can be seen as relying on the existence of complex switches at the sub-cellular level: for Pribram [23] through teledendrons and dendrites; for Hameroff and Penrose [16] through microtubules; for Eccles [8] at a synaptic level.

The MP at the level of assemblies of neurons has already been singled out as such by Edelman [9] under the name of Degeneracy Principle (by analogy with the 'degeneracy' of the genetic code in which the same aminoacid can be coded by two different codons).

3.3 Memory

The Memory Mem develops through the collective action of the different CRs and their interplay of strategies. It consists in a flexible, though stable enough, archive for the various experiences of the MES. It has a central role, since a record (i.e., an object in it) may be perceived by different CRs through different aspects and with different decompositions, with lower CRs handling only some particular attribute (e.g., color, size,..) of the object recorded.

Let us show how an external stimulus Q_M will be recorded. In its landscape, a lower CR, say E, sees only aspects r' of a sub-pattern R of the receptors corresponding to a particular attribute (e.g. color); R is memorized by the formation of a colimit M' which represents the E-record of the event, possibly seen through its aspect m' in the landscape of E. In the same way a higher CR which sees aspects r_M of a larger pattern R_M of receptors will form its record M of Q_M , seen through its aspect m: M ® C_M in the landscape of the CR. Because of the interplay of strategies, M is also the colimit of the records (such as M') formed by lower CRs which the higher CR controls.

Later if the same stimulus is met anew, the record can be accessed through the landscapes of the various CRs, and possibly recalled via any of its different ramifications.

The records of external stimuli (transmitted by sensorial receptors) and their links form a sub-system of Mem, called the empirical memory, and denoted by Emp.

The strategies used and their results are also recorded, and they form a sub-system of Mem called the procedural memory, denoted by Strat. It contains the records of the different behaviors of the animal.

3.4 Semantics

The neural system of a more complex animal is able not only to memorize its experiences of all nature, but also to classify their records into invariance classes. To model this classification process in the MES of neurons, we assume that the successive complexifications also add limits, called CR-concepts, classifying invariance classes of records with respect to some of their attributes. Such a classification requires an internal reflection, with lower CRs effecting a 'pragmatic' classification, and higher CRs able to 'interpret' this classification and give it a representation.

Indeed, let E be a lower CR corresponding to some attribute (e.g. color); an external stimulus Q_M (or its record M) activates a pattern P of agents of E, called the E-trace of M. Two items are 'acted' as equivalent by E if they have the same E-trace; for instance, in a color-CR, it is the same pattern of receptors which is activated by all blue objects.

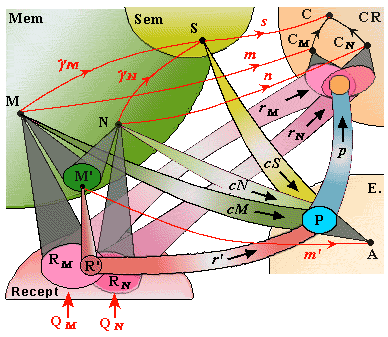
But this classification takes its meaning only at the level of a higher level CR with a longer period, able to overview that different records have the same E-trace (same color), and to store it in the memory under the form of an object (the color blue) representing the whole class of these records. This object, called the E-concept of M, is constructed as the (projective) limit* S of P, seen by its aspect s: S ® C in the landscape of the CR. There is a link g_M from M to S, modeling the fact that M is one instance of the concept S.

The activation of the concept S may activate either of its instances, with possibly a balance from one instance M to another one N. Moreover the sole activation of M may activate its concept S which may then activate another instance N of S, thus starting a balance between M and N.

The CR-concepts, with respect to the various CRs, are at the root of the formation, through successive complexifications, of general concepts, constructed as iterated limits of patterns of CR-concepts. First concepts simultaneously classifying several features are formed (as a blue triangle), and then more abstract concepts obtained as limits of patterns of such 'concrete' concepts linked by complex links.

A concept can be thought of as an abstract prototype for a class of objects with a 'family resemblance' (in the sense of Wittgenstein); it does not necessitate the existence of a language.

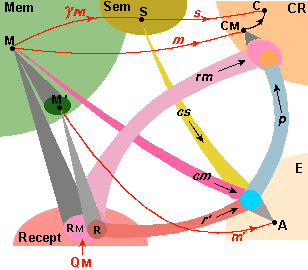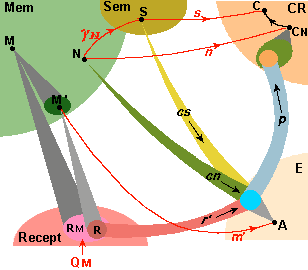
The later re-activation of a concept relies on a double indeterminacy: balance between different instances of the concept, then switches between different ramifications of the instances. Remark that these two operations of balance are of a somewhat dual nature: The balance realized by a complex switch between two patterns corresponds to an alternative between two non-equivalent decompositions of the same higher object; the selection of one of them can be seen as a classical analog of the collapse of the wave function of a (quantum) superposition. The balance between 2 instances of a concept corresponds to an alternative between two different presentations of the attribute(s) they have in common; it can be seen as a classical analog of a non-localization process, the attribute(s) being presented differently in both. (It is these extensions of quantum properties that Aerts et al. [1] single out.) In the first case, the two alternatives merge at the higher level; in the second, they appear by dissociation of a common base.

The concepts and their links form a sub-system of Mem, called the semantic memory and denoted by Sem.

The development of a semantic memory adds flexibility to the interplay among the strategies of the CRs. Indeed, concepts representing invariance classes of strategies in the procedural memory Strat will be formed, and the choice of a strategy by a higher CR will be done under the form of such a concept, instead of a specific strategy of its invariance class. It adds a new degree of freedom in the formation of the global strategy effectively realized on the system at a given time, since the interplay among the strategies may select among the strategies of the invariance class chosen by a CR the best adapted one, taking into account the strategies relayed by the other CRs. For instance, the command of seizing an object will be modulated depending on the object to hold.

3.5 The Archetypal Core

We have seen that the memory Mem is divided into 3 sub-systems with links between them: the empirical memory Emp, the procedural memory Strat, and the semantic memory Sem where the records are classified into concepts. Now we will draw a distinction among records depending on the difference of their strength, thus displaying 2 other sub-systems of Mem : the archetypal core AC and the experiential memory Exp.

The records of patterns which are activated more often and during a longer period, from birth on (for instance stable aspects of the environment in contrast to more variable ones, deep feelings,…) and their links form a particular sub-system of the memory, called the archetypal core, denoted by AC. It develops to integrate the main sensorial, proprioceptive, motor experiences, …, with their emotional overtones, and connects them in patterns with strong links, quickly activated and gradually strengthened. It represents a personal 'affective' memory of the animal, his body, his experiences, his acquired knowledge, be it pragmatic, social or conceptual and the basic strategies associated to them.

The archetypal links may be autonomously activated, so that a whole sub-system of AC is activated as soon as a small part of it is stimulated. And whatever are the variations of the stimuli this self activation along the links of the archetypal core can be maintained long enough. It is generated through a sequence of loops based on balances between the various instances of its concepts and complex switches among the ramifications of these instances (in the neural system, it relies on the thalamo-cortical loops). For instance a record in AC (say the blue sky) is also archetypally linked to other records not only of perceptions or of motor processes but also of internal states and emotions (sun, heat, well-being, swimming …).

Some records represent experiences which are significative enough to have strong links toward the archetypal core, possibly without belonging to it; with their links they form a sub-system of Mem, called the experiential memory, denoted by Exp (to be related to the value-category memory of Edelman [9]). Its objects may represent external stimuli as well as internal stimuli, behaviors or strategies, or an association of such.

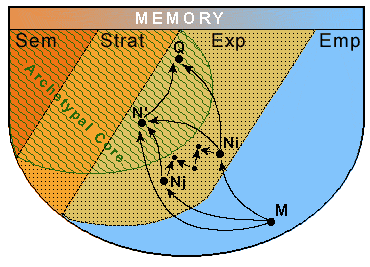
Each record N in Exp is linked to a 'nearest' object of AC in the sense that the activation of N re-activates the 'archetypal' experience most closely linked to N, which then diffuses to other experiences linked to this one in the AC, and the activation thus spreads into loops which remain self-generated for a long time and may extend to closer areas. Formally, AC is a reflective* sub-system of Exp in the sense that for each N there exists a link from N to an object of AC through which any other link from N to an object of AC factors uniquely.

By successive complexifications directed by the net of CRs, the memory develops and is extended by the formation of new colimits M which become empirical or procedural records. Such a record is linked by a cluster to a pattern of related experiences N_i in the experiential memory. Formally, Exp is a final* sub-system of Mem. Since, as said above, the archetypal core is a reflective sub-system of the experiential memory, each experience N_i activated by M also recalls its closest archetypal record N', from which the activation diffuses to other records Q along the different links of the archetypal core.

The organization of the memory is further enhanced by the recognition by a CR of a semantic identity between records which makes it possible to classify them into concepts. If the links from a record to Exp become strong enough, the record will become integrated in the experiential memory, and possibly later on in the archetypal core. While only a part of the empirical memory becomes experiential, the entirety of Strat and Sem develop into sub-systems of Exp. And, at least at the beginning, the majority of the experiential memory is progressively integrated in the archetypal core, in priority the records coming from internal stimuli.

For instance, the archetypal core of an infant already contains a holding reflex. It will progressively develop in a well directed sensori-motor strategy for seizing an object: a visual CR will learn to recognize some objects (in Emp), an 'emotional' CR will recall the pleasure (in AC) of seizing some objects, a motor CR will recall how an object has been seized (strategy in Strat). When the baby recognizes an object, the links between these different records are activated, with balances between the different instances of the concepts associated to them, so that these CRs will cooperate by the interplay among the strategies to try to seize the object. The baby will learn to adjust his motions to the size and form of the object, and this will be memorized through the formation of a colimit representing the particular act, then formation of an archetypal concept of the sensori-motor 'seizing an object' behavior.

3.6 Extended landscape. Consciousness

Building on the above results and those of Ehresmann & Vanbremeersch [13] we propose to characterize consciousness as a 3 parts process relying on the development of the archetypal core which, always in the background, acts as a referent and as a filter: (i) formation, through balances and switching, of an extended 'holist' landscape which allows to operate (ii) a retrospection toward the past to find the causes of an event; and (iii) a prospection toward the future to find the best adapted strategies. The degree of consciousness will depend on the development of the archetypal core: more developed is AC and more extended will be the constructed landscape, thus allowing for a longer retrospection in the past and for the selection of more long term strategies. These processes are defined as follows:

Let us suppose that, within the empirical background noise, a new significant event appears which has no automatic response (e.g., a fracture in a higher landscape). It immediately determines an increase of awareness (activation of the reticular formation) which starts a semiotic search in the memory through the CRs of all levels (color, shape,…) up to semantics to try to recognize it, through a process of balance and switching along the complex links. Indeed, among the records thus activated some form sub-patterns admitting the same semantic projection as archetypal records or non-archetypal experiences in Exp. It follows that the landscapes of higher CRs receive, for one C1 aspects coming from the archetypal core, for another C2 aspects of the experiential memory and of the empirical memory. As the 2 CRs are bound by the perspectives of links between Emp, Exp and AC, the CRs form together an alternate, ambivalent perception of the experience and of the empirical stimulus. A still higher CR receives an alternative of aspects, at different intervals, of these empirical, experiential and archetypal records and of their various ramifications. Each CR acts at its own temporality; the balances of archetypal origin by definition are more remanent, being reactivated over a greater duration than those of the empirical memory.

(i) In this way, higher CRs connect the landscapes of the different CRs they control, taking into account the specific experiences of the subject as they are reflected by the archetypal core. Thus is formed a 'holist' extended landscape, whose objects are the temporal perspectives of the event and of its internal reflections, in particular in AC (to be compared with the "Global workspace model" or "theater" of Baars [2]). Physiologically its construction relies on the existence of functional loops between various parts of the brain, which form what Edelman ([9], p. 100) calls the "consciousness loop".

(ii) A retrospection process, based on a series of balances and switching, will be operated on this extended landscape; it uncovers lower levels of the near past to find, by abduction, the possible causes of the present event.

(iii) Then a prospection process (oriented toward the future) will select long term strategies for several steps ahead, to respond in the most adaptive way. This is accomplished through the formation, inside the extended landscape, of virtual landscapes, with the help of the archetypal core and of the whole memory, in which successive strategies (selected as concepts) can be 'tried' without material cost for the system.

In this model, the evolution of the archetypal core and of consciousness relies on the existence of balance and complex switches which results from the MP. It takes into account the whole experience, be it perceptive, behavioral or emotional (the role of emotions has well be emphasized by Damasio [6]), with an integration of the temporal dimensions. The permanence of the archetypal core, or at least its very progressive modification, could be the basis of the notion of self. And for the "hard problem", the qualia could correspond to perspectives of objects of the archetypal core in the extended 'conscious' landscape.

The evolution of such a consciousness gives a selective advantage, since it allows to search for the causes of an event instead of just reacting to its effects. And by programming on the long term, more efficient strategies may be devised, less constrained by the immediate concerns. It does not need language (higher animals may have such a consciousness).

But language allows for more efficient processes, because complex information (higher level cat-neurons) can be stored in the more compact form of a word, and thus more operations can be effected on it. The consequence is the development of a richer 'algebra of mental objects' (in the sense of Changeux [4]) and of higher order cognitive processes, depending heavily on social interactions, through communication and education.

4 MATHEMATICAL DEFINITIONS

• A category is a(n oriented multi-)graph on which a composition rule has been given which associates to 2 successive arrows: f: N ® N' and f': N' ® N" an arrow ff': N ® N". This composition must satisfy two axioms:

(i) Associativity: To each path of length n is associated a unique composite, independent from its 2-2 decomposition; (ii) Identity: to each vertex is associated a closed arrow whose composite with any other arrow gives this arrow. The vertices of the graph are called objects of the category, and its arrows morphisms (or links).

A partial functor from a category K to a category K' is a map F from a sub-category C of K to K' which associates to each object N of C an object F(N) of K and to each morphism f: N ® N' of C a morphism F(f): F(N) ® F(N') so that the composite ff' be applied on the composite of the images of f and f' .

• A pattern (or diagram) P in a category is formed by a family of objects N_i of the category and some distinguished links between them.

A collective link from P to an object N' is a family of links f_i: N_i ® N' correlated by the distinguished links of the pattern in the sense that, if g is a link in P from N_i to N_j, we have gf_j = f_i .

P has a colimit (or inductive limit) if there exists an object N such that: (i) ("universal property") there exists a collective link (c_i) from P to N; and (ii) each collective link (f_i) from P to any object N' binds into a unique link f from N to N', so that f_i = c_if for each i.

If a pattern has a colimit, it is unique (up to an isomorphism).

The sum (or coproduct) S of the family of objects (N_i) is the colimit of the pattern formed by these objects with no distinguished links. If this sum S and the colimit N of P both exist there is a canonical 'comparison' morphism from S to N.

A (projective) limit of a pattern P in the category K is a colimit of the opposite pattern in the category opposite to K (obtained by changing the direction of its morphisms).

• If P and P' are patterns, a cluster from P to P' is a maximal family of links from objects of P to objects of P' such that: (i) Each object of P is linked to at least one object of P', and if it is linked to several its links are correlated by a zig-zag of distinguished links of P'. (ii) The composite of a link of the cluster with a distinguished link of P or of P' is also in the cluster.

If P and P' admit colimits N and N' respectively in the category, a cluster from P to P' binds into a unique link from N to N' called a (P,P')-simple link.

The composite of two simple links binding adjacent clusters is still a simple link: if f is (P,P')-simple and if f' is (P',P")-simple, then their composite ff' is a (P,P")-simple link. But a (P,P')-simple link might not be (Q,Q')-simple for other decompositions Q of N and Q' of N'.

Two decompositions P and Q of an object N are equivalent if there exists a cluster between P and Q which binds into the identity of N, so that this identity is a (P,Q)-simple link. Otherwise, we say that P and Q are non-equivalent decompositions of N.

A complex link is a composite of simple links binding non-adjacent clusters, with complex switches between the different decompositions of the intermediate multifold objects.

• An Evolutive System K is the data of: a time-scale T, which is a discrete or not, finite or not, part of the positive reals; a category K_t for each t of the time-scale and a partial functor called transition from K_t to K_t' for each t < t'.

A sub-system L of K is an evolutive system whose time-scale is a subset S of T and whose category L_s is a sub-category of K_s for each s in S.

L is a final sub-system of K if S = T and if for each object N of K_t there exists a cluster from the pattern reduced to N to the pattern represented by L_t. It is a reflective sub-system if S = T and if, for each object N of K_t there exists an object N' of L_t and a morphism k from N to N' such that each other morphism from N to an object of L_t has exactly one factorization through k.

• In a hierarchical system, the MP can be strengthened as follows: (i) There exist objects of level n+1 which are n-multifold in the sense that they admit at least two non-equivalent decompositions into patterns of level n. (ii) An object of level n can belong to several patterns admitting different colimits of level n+1.

• The actual landscape of a CR at a time t is constructed as follows. An object B of the system has an aspect b for the CR if b is a link from B to an agent of the CR. Two aspects are in the same perspective if they are correlated by a zig-zag of links between agents of the CR (so that they 'transmit the same information'). The landscape L is the category whose objects are the perspectives coming from objects of a level adjacent to the level of the CR and which last during the present step of the CR (its length is determined by the time-scale of the CR. The morphisms come from morphisms in the system, so that there is a 'distortion functor' from L to the system (it measures how deformed are the partial information of the CR).

A step for the CR consists in forming its actual landscape L, selecting a strategy on it (with the use of the Memory), and transmitting the corresponding commands to the effectors. The anticipated landscape L' at the next step should be the complexification of the landscape with respect to this strategy. The CR can then evaluate if the objectives have been attained by a comparison functor from L' to the landscape effectively obtained at this next step.

CONCLUSION

In this paper, we have proposed a mathematical model, based on category theory, for the emergence processes, which lead progressively to the evolution of the universe from the quantum level up to the level of complex organisms and even conscious beings. This evolution is described as a succession of complexification processes, in which patterns of interacting objects are aggregated into new higher objects taking their own complex identity (represented in the categorical model by the colimit of the pattern). What we try to explain is in what sense such a new object N has emerging global properties compared to the properties of the pattern P it binds, i.e., compared to the objects of its decomposition P.

At the atomic level, an atom binds together the pattern formed by its electronic configuration in atomic orbitals. But Quantum Physics asserts that the atom has several such configurations related to different energy levels; we say that it is a 'multifold' object. We claim that this property is the key of the emergence problem.

Indeed, more generally N' is a multifold object if it admits a decomposition into 2 non-equivalent patterns, say P' and R', so that it can 'switch' between these two decompositions; this fact cannot be recognized just by considering P' and R', but depends on the whole structure of the lower level to which they belong (the quantum level if N' is an atom). The existence of multifold objects entails the existence of 2 kinds of links: a (P,P')-simple link f from N to N' just binds together a cluster of links between the objects of the decompositions P of N and P' of N', and thus does not represent 'new' properties with respect to the lower level. But there also exist complex links which are composites of simple links binding non-adjacent clusters, e.g., a link from N to N" which is the composite of f with a (R',P")-simple link f' from N' to N", where N' is multifold; this link represents new emerging properties at the level of N and N", since it depends not only on the constituents of N and N" of the lower level (reductionism) but on the whole structure of this lower level through the existence of a switch between P' and R'.

By a categorical reasoning, we prove that, if a system has multifold objects (we say that it satisfies the multiplicity principle), so do all its successive complexifications, and they lead to the formation of objects of increasing orders of complexity connected by complex links that represent, at each level, new emerging properties (in the above sense). As the quantum system satisfies the MP, it follows that the MP is satisfied by all the natural systems which have evolved from it or its sub-systems by successive complexifications.

In Section 3, we have applied this result to some autonomous anticipatory systems, such as biological and more specially neural systems, modeled in the categorical frame by a Memory Evolutive System. Successive complexifications of the category of neurons lead to the emergence of higher and higher cognitive processes. Recalling some of our previous papers, we describe the development of a general memory, and of a classification of its records in a semantic memory. And we proceed to study how consciousness may arise.

The new idea here is that the emergence of consciousness relies on the formation, from birth on, of a sub-system of the memory, the archetypal core, formed of multifold objects, which integrates the main sensorial, proprioceptive, motor experiences, …, with their emotional overtones, and connects them in patterns with strong links, quickly activated and gradually strengthened. A new event starts a semiotic search in the archetypal core and in the records linked to it, through balances between their different decompositions. It leads to the formation of a 'holist' extended landscape, in which are effected: a retrospection process toward the near past to find the causes of the event; and a prospection process to select long term strategies for the future.

Thus consciousness appears as a process which takes into account the whole experience of the subject, with its multiple aspects, integrates the temporal dimensions, and gives him evolutive advantages by allowing for more adapted responses. It relies on the quantum level, not directly as several authors have suggested, but in a long series of steps, through the progressive emergence of more and more complex objects during the evolution, emergence which, as said above, relies on the satisfaction of the MP in the quantum system, which entails its satisfaction in the complexifications deduced from it.

Acknowledgments

We are grateful to Jerry Chandler, George Farre and Brian Josephson for stimulating exchanges on related problems.

REFERENCES